For the EOQ equation, demand is the number of units your business will purchase in a given timeframe, typically one year, reflecting the number of units you expect to sell.
Thus, ordering cost might include freight and the time your company’s purchasing team spends researching and placing the order.
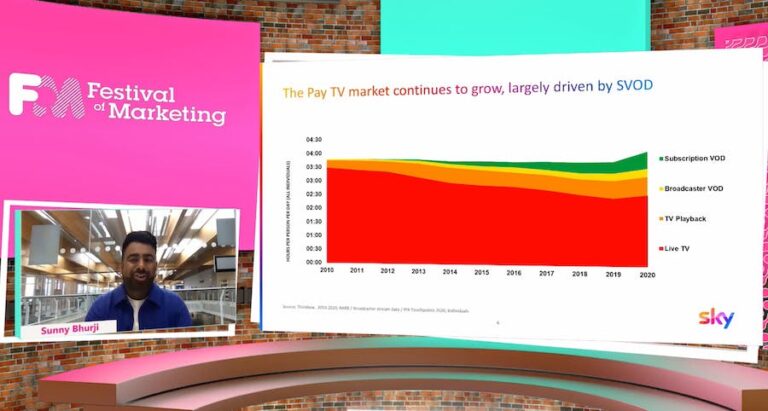
This balancing act is what the EOQ tries to solve, using an equation that identifies ordering cost, demand, and carrying cost.
Having identified the ordering cost, the demand, and the carrying cost per unit, we are ready to calculate the EOQ or optimal order size, which is Q* in our equation.
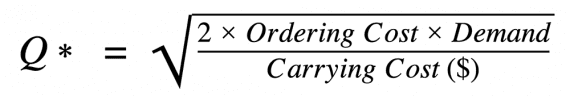
The economic order quantity or optimal order size is the square root of two times the ordering cost times the demand for a given timeframe divided by the carrying cost per unit.
In this case, it would make the most sense to order 1,000 units ten times throughout the year.

For example, imagine a DTC brand that manufactures its product in Taiwan. With each production run, the brand sends a quality engineer from its office in Los Angeles to the facility in Taiwan. The trip is part of the ordering cost.
The EOQ demand is the number of units your business will purchase for some timeframe, such as one year.

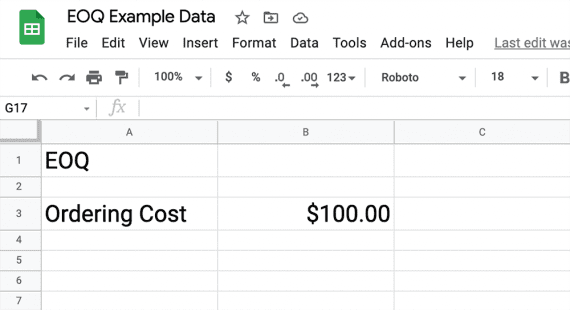
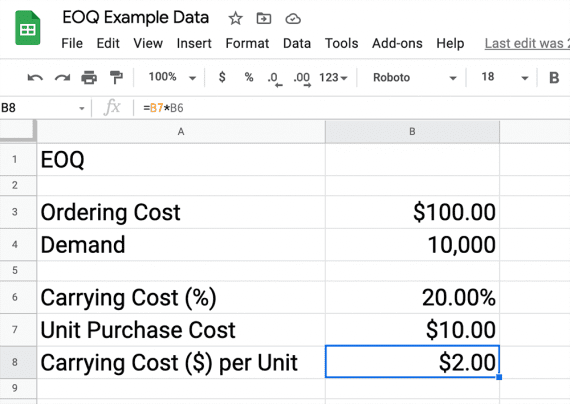
Imagine that the ordering cost of a product is 0. We can place this number in a Google Sheet.
Inventory carrying costs typically include the costs of capital, storage, servicing, and risk.